8
It turned out that the President of the Royal Society of England, Alain Hodgkin, found out almost everything about this generator. His work was a real success story of physics in biology (Hodgkin, 1937), for which he was awarded the Nobel Prize.
Phase theory prevailed in Soviet physiology at that time. It was clear that the change in the sign of a potential during the generation of a nerve impulse could not be explained by the phase properties of protoplasm. And I came up with a phase-membrane hypothesis that was in compliance with the already set experiments (Liberman, 1961). Afanasy Troshin, who was a proponent of phase theory, included the presentation of this hypothesis in a talk he gave in England and in his book. He told me that it was this talk that prompted Alain Hodgkin's experiments with perfusion of the giant squid axon, which killed the phase-membrane hypothesis outright: ordinary nerve impulses were generated by a membrane devoid of protoplasm. Fortunately, I had wonderful colleagues Aleksey Babakov and Lev Ermishkin, and we began to simulate a nerve impulse on artificial phospholipid membranes (Babakov et al., 1966).
Neuromuscular synapse
The British scientists Paul Fatt and Bernard Katz found that neurotransmitters are released from nerve endings in portions (Fatt, Katz, 1952). At rest, each portion causes a small change in the potential difference on the muscle fiber membrane, which is called "miniature potential". Portions of acetylcholine are released spontaneously at rest. A nerve impulse increases the average frequency of their release thousands of times. Many simultaneously emerging miniature potentials merge to form a synaptic potential. When using the same apparatus for counting alpha particles, we learned to divert miniature potentials from the neuromuscular junction of a frog; it remained to solve the question of why an incoming nerve impulse accelerates the adhesion of synaptic vesicles to the outer presynaptic membrane of the nerve ending.
At first, it seemed that the reason was a decrease in the potential difference across the membrane caused by the arrival of a nerve impulse.
Indeed, in a normal saline solution, all kinds of influences that reduce this potential difference increase the frequency of miniature potentials. But if calcium ions are removed from the ambient solution, then an increase in frequency does not occur, despite the arrival of a nerve impulse.
But the most incomprehensible thing was the sharp increase in the frequency of release of quanta with an increase in the concentration of any non-penetrating ions or neutral molecules (for example, sucrose) in an external saline solution. Zhanna Bliokh, Irina Glagoleva and I (Blioch et al., 1968) managed to sort out this confusing situation. We had a neuromuscular preparation of the frog pectoral muscle, which controls skin movement and is convenient for studying miniature potentials. This preparation consisted mainly of a single layer of muscle fibers, to which the nerve approached perpendicularly and branched out in the form of an arabesque tree, sending nerve endings to single muscle fibers. On a simple paraffin plate, on which the frog's neuromuscular preparation was usually attached, the transparent fibers of this pectoral muscle were almost invisible. But on the advice of Lilia Tsofina, who found this preparation for us, the addition of crushed activated carbon to the paraffin made it possible to perfectly see muscle fibers and nerve endings. With this preparation, it was possible to obtain miniature potentials, the quality of which was appreciated by the great Bernard Katz himself when he visited our laboratory.
We proceeded from a simple physical idea: for the vesicles to adhere to the outer membrane of the presynaptic fiber, its surface electric charge must be reduced.
Calcium ions can do this. Experiments on the adhesion of artificial phospholipid membranes were staged by Valery Nenashev. In solutions with calcium, the adhesion of such membranes occurs much faster.
9
We reasoned that each nerve impulse causes not only depolarization of the presynaptic end, but also the entry of calcium ions into the end of the nerve fiber. Therefore, in a solution without calcium, there is no synaptic transmission. At the same time, this hypothesis explained the dependence of the frequency of miniature potentials on osmotic pressure.
This effect had gone unexplained for a long time, since osmotic pressure does not affect the propagation of a nerve impulse in any way. The idea of a decrease in the surface charge immediately explained the effect of osmotic pressure: an increase in the concentration of ions or neutral molecules in the external solution leads to the fact that water leaves the cell and, therefore, the intracellular concentration of cations, including calcium, increases. We sent the paper to Bernard Katz, who had visited us before and observed with pleasure his miniature potentials in our lab.
The paper publication was delayed for a long time. Paul Fatt translated it into English. While our paper was with them, Bernard Katz reported that it was possible to conduct direct experiments with the introduction of calcium ions into the giant synapses of squid. In these synapses, the thickness of the presynaptic fiber reaches a millimeter.
He asked us to refer to these experiments, which, in my opinion, cannot be reproduced, since calcium is absorbed by mitochondria on the way to the outer membrane. By adding uncouplers of oxidative phosphorylation, Irina Glagoleva and Zaur Khashaev were able to release calcium from mitochondria inside the nerve endings.
It turned out that all substances that remove the electric field from the mitochondrial membrane sharply increase the frequency of release of acetylcholine quanta (Glagoleva et al., 1970).
Electrical energetics of a living cell
At the seminar of Academician Israel Gelfand, Vladimir Skulachev told me that Peter Mitchell, an Englishman whom no one understands, entered the field of bioenergetics. Skulachev pointed me to Peter Mitchell’s paper (Мitchell, 1961). I am lucky that I do not know much English, otherwise I would always be overwhelmed by the flow of scientific literature. The paper was translated for me by my colleague Lilia Tsofina with whom I have been working for about fifty years. She is a graduate of the Department of Animal Biochemistry of the Moscow State University Faculty of Biology, and with her we understood Mitchell's then barely comprehended new ideas about the important properties of the mitochondrial membrane. After the first sentences of the paper, I thought it concerned the potential difference on the mitochondrial membrane. However, the author thought that only a pH gradient would appear. He was unfamiliar with the works of Alain Hodgkin. Together with Lev Krishtalik, Vyacheslav Markin and Vasily Topaly, we began testing Peter Mitchell's idea that uncouplers of oxidative phosphorylation are proton carriers (Liberman and Topaly, 1968; Markin et al., 1969).
Simultaneously, together with Lilia Tsofina, we measured the membrane potential of mitochondria (Fig. 2), (Liberman et al., 1969). Lilia Tsofina, Pulat Isaev and I also measured the membrane potential of bacterial photoparticles (Fig. 3).
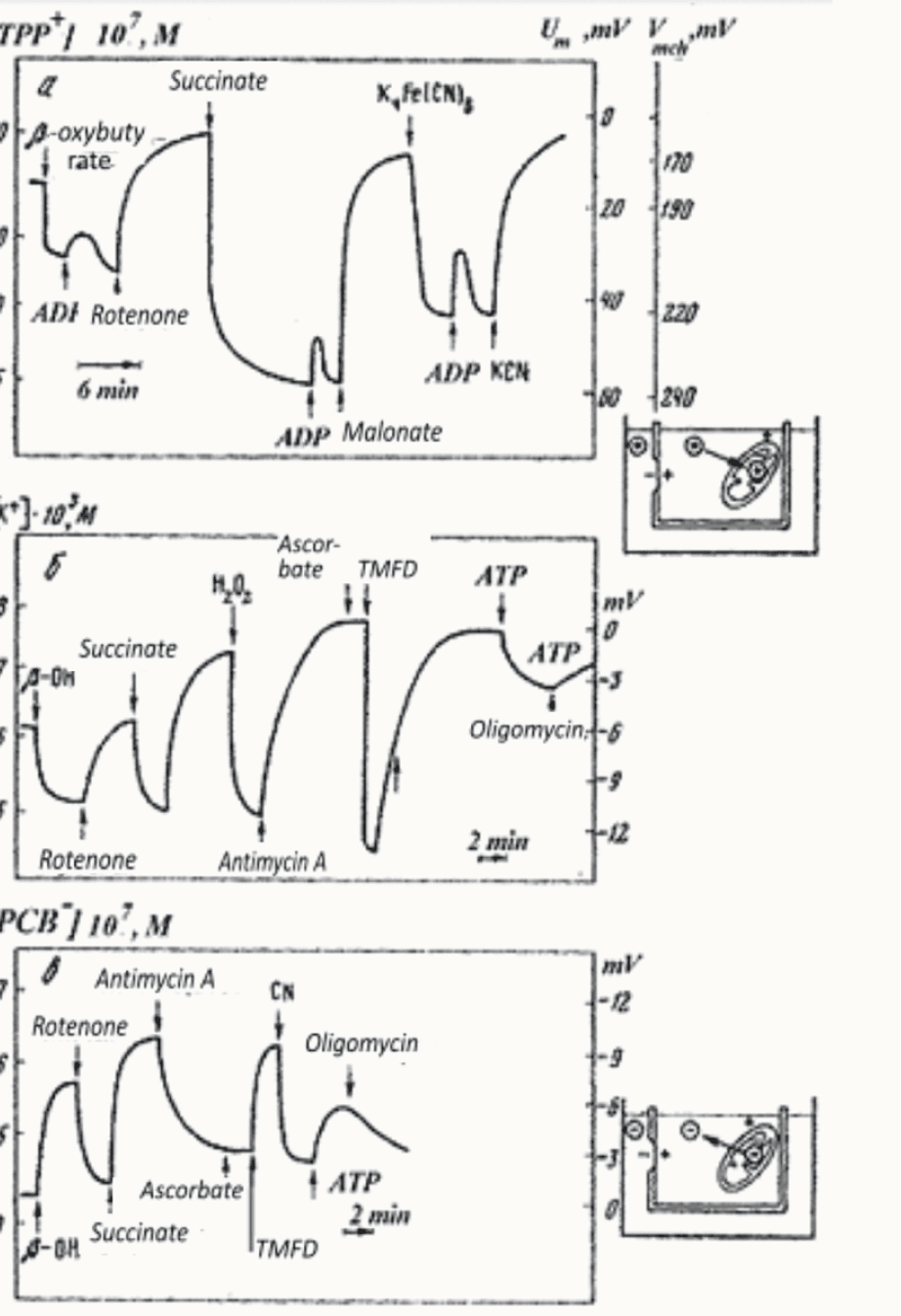
Figure 2. Membrane potential of mitochondria study (Liberman and Tsoflina, 1969).
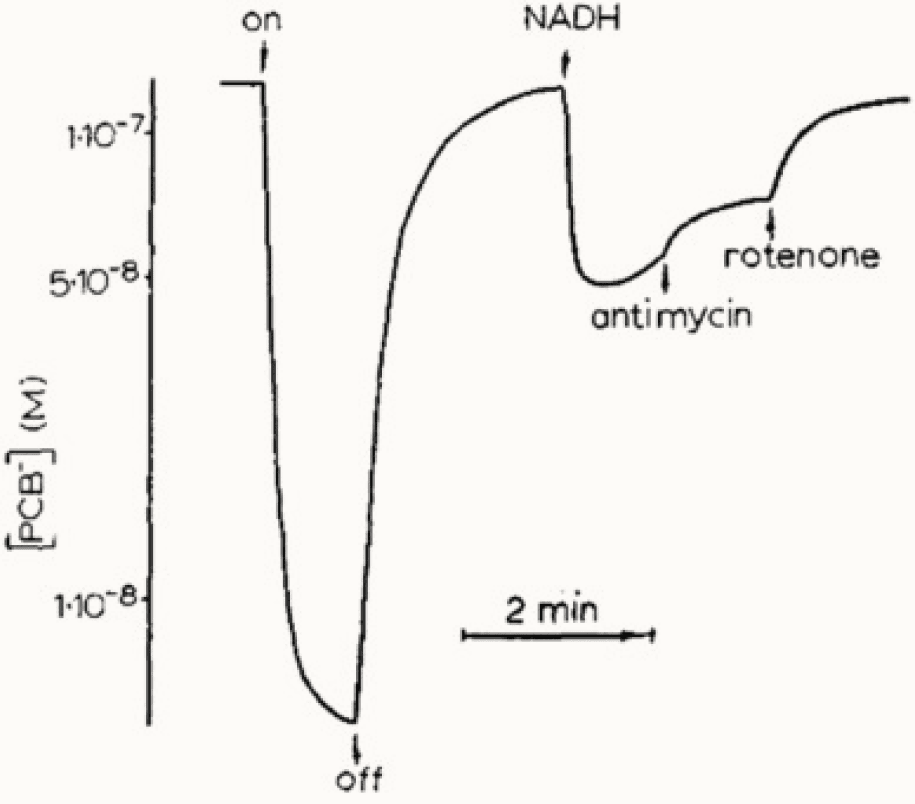
Figure 3. Transport of phenyl dicarbon-decaborate– (PDCDC–) in chromatophores of photosynthetic bacteria. Penetrating ion concentration is shown at the vertical axis (Isaev et al., 1970).
All these measurements were carried out using the penetrating ion method developed by Vasily Topaly and myself (Liberman and Topaly, 1969)
We worked on mitochondria, which were specially isolated for us by Elena Pinus and Inna Gorskaya. Valdur Saks made submitochondrial particles, and Viktor Samuilov made photoparticles. Vladimir Skulachev (a talented manager who actively participated in research, challenged us and kept us informed of the achievements of world science) created a wonderful spirit in the molecular biology building of the Moscow State University Faculty of Biology, where parts of this work were carried out.
Marina Vladimirova figured out how the proton channel works in the mitochondrial membrane. She personally isolated the submitochondrial particles, stripped off ATPase from them, and measured how the membrane potential changes after the addition of oligomycin. This experiment allowed us to ascertain that this inhibitor actually closes the proton channel of ATPase (Grinius et al., 1970).
In 1975, V.P. Skulachev, L.M. Tsofina, A. Yasaitis, and I were awarded the USSR State Prize for a series of papers on the experimental proof of the new role of mitochondrial membrane proteins in charge transfer during the process of ATP synthesis.
However, soon my role in bioenergetics came to naught thanks to the appearance among us of Lel Drachev, a real experimenter (Drachev et al., 1974).
I had to continue the path to Chaimatics. On this path, Anatoly Zilbershtein supported me all the time and served as a “workhorse”.


Efim Liberman (sitting left), Svetlana Minina (sitting right), Vasily Topaly (standing left), and Anatoly Zilbershtein (standing right) in the 1970s.
10
chaimatics
Chaimatics
Discovery of links between the biology, physics and mathematics, and founding a new area of studies focused on computations in living systems are his life achievements. Efim Liberman gave the name of “Chaimatics” to this new area of science
I
DNA is the text of a code written for molecular computers of living cells. The notion of “Text” is intrinsically opposite to a random sequence of symbols, and it can exist only inside the system of language. In this case, it is a genetic language, which is isomorphic to a natural language
II
Computations conducted in a living cell are real physical actions, and free energy and time must be spent for completing them. As all living organisms are comprised of cells, this statement is applicable to any control processes implemented in the biosphere
III
Molecular computations are limited by the microscopic scale of a cell and inevitable impact of the computations on formulation of a problem begin solved. The Chaimatics grew from the recognition of the computation reality as the quantum mechanics grew from the recognition of the measurement reality.
IV
A cell creates а quantum computing tool for solving complex problems. This tool utilizes hypersound quanta, and uses the cell cytoskeleton as the computing environment. In such a computer, a price of elementary computation converges to the physical limit, which is Planck’s constant
Chaimatic's statements are simple, but they require a change in the traditional vision, rooted in scientific practice
Read a book
Chapter I
The journey of life in science
chaimatics
